A point source is the theoretical ideal; why, then, do almost all loudspeakers sport two, three or even more drivers spread out over a three-quarter meter baffle? Karl-Heinz Fink, mastermind and developer behind the products of FinkTeam, Epos and many other brands, sheds light on the basic principles and problems of crossover design for us.
The human ear is an amazing organ: with the help of a single membrane, it is capable of capturing a frequency range from 20 to about 20,000 hertz – that’s ten octaves! Speaker drivers would break out in a sweat at the mere threat of such a challenge. That’s exactly why almost all developers spread the load across multiple drivers optimized for narrower bands. I write “Almost all developers” because full-range speakers are a thing – and this approach has some merit, because as Karl-Heinz Fink explains to me at the very beginning of our conversation, “The best crossover is no crossover.” With this renunciation, however, you force some balancing act upon yourself that are nigh impossible to master. If a driver is to cover the entire audible range, it can essentially neither be large enough nor small enough. Since diaphragms emit wavelengths smaller than their own diameter in a directional manner, small drivers are advantageous in the treble since they do not beam too much at high frequencies and thus enable a flat, even radiation pattern. However, as the frequency decreases, more air must be shoved around with each stroke, and diaphragm area is indispensable to do this.
In addition, a full-range driver cannot simply be left running unfiltered. The reason for this is the so-called “baffle step”: while wavelengths shorter than the width of the baffle are radiated forward by the speaker, longer waves (i.e. lower frequencies) “wrap” around the cabinet. In the low frequencies, therefore, twice as much air volume must be supplied with sound pressure than at the top. If a linear signal is fed to the loudspeaker over the entire bandwidth, the sound pressure is halved in this range, which corresponds to a drop in SPL of 6 decibels. The resulting “step” in the frequency response has to be smoothed out if a well-balanced loudspeaker is to emerge in the end. And speaking of cabinets: They, too, act as filters, just acoustically and not electrically. This becomes obvious at the speaker’s lower cutoff frequency: In combination with the driver, the cabinet forms a high-pass filter that causes the bass level in a closed system to drop below the cutoff frequency at 12 decibels per octave. In bass reflex speakers, the frequency is lower, but the drop below it is steeper at 24 decibels per octave. Midrange and tweeter drivers also exhibit such filtering functions in their respective enclosures. “When choosing a topology, it’s important to keep in mind that the acoustic and electrical effects added together determine the final result,” Fink explains.
Frequency what?
So in the end, there’s little getting around the necessary evil of a crossover – so how is it constructed? The essential components are high, low and bandpass filters, each implemented with a combination of capacitors and coils. In most crossovers, resistors are also built in, for example to adjust the level of the individual drivers to each other. A high-pass filter, i.e. a filter that allows only high frequencies to pass, consists in the simplest case of a capacitor that sits in series between the amplifier output and the tweeter. Its capacitance can be used to set the frequency below which the filter effect kicks in – the higher the capacitance, the lower the crossover frequency. The result is a “first order” filter. Such a filter lowers the signal level by 6 decibels per octave, i.e. with each halving of the frequency, the signal amplitude is halved. If you add an inductor to ground after the capacitor, you get a second-order high-pass filter that operates with twice the slope steepness: The amplitude decreases to a quarter with each octave, i.e. by 12 dB. By adding a second capacitor behind the coil, we get a third-order filter that separates at 18 dB/oct, and so on.
If we replace all the capacitors with coils in this setup, and vice versa, the high-pass filters become low-pass filters that operate according to the same laws: A single coil filters out high frequencies with a slope of 6 dB/oct. The decisive characteristic value in this context is called inductance, where larger values likewise lead to lower crossover frequencies. Analogous to the high-pass filter, an inductor and a capacitor result in a second-order low-pass filter (12 dB/oct.), another inductor in a third-order filter, and so on.
Finally, the combination of the two aforementioned filters forms what we call a bandpass: A network that uses a low-set high-pass in conjunction with a high-set low-pass and allows the frequency band in between to pass, which then supplies signal to the midrange driver of a three-way loudspeaker, for example.
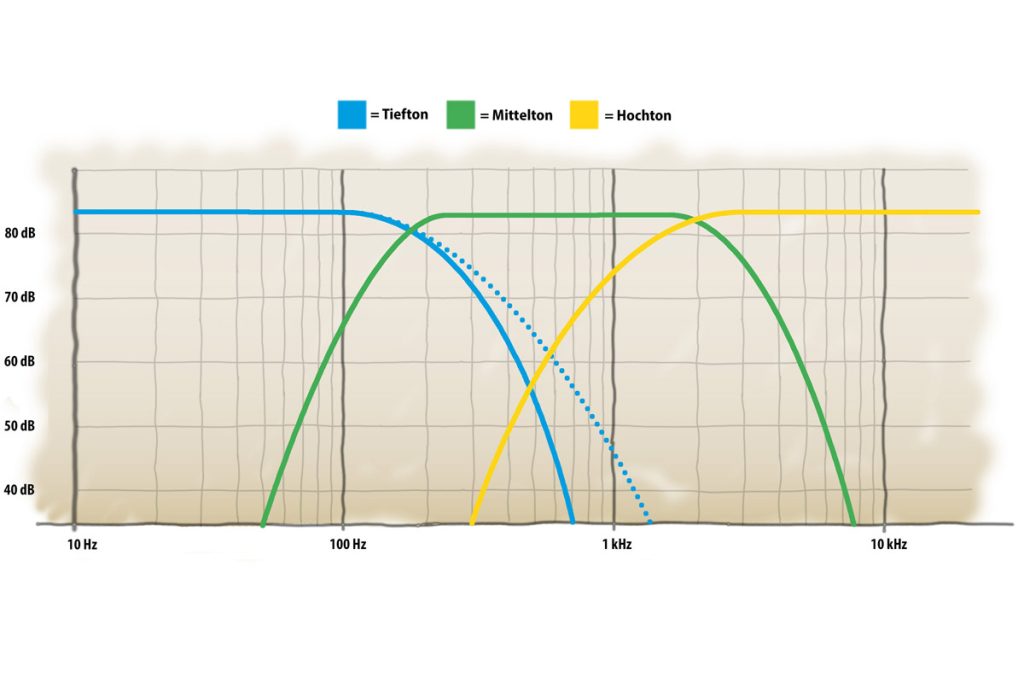
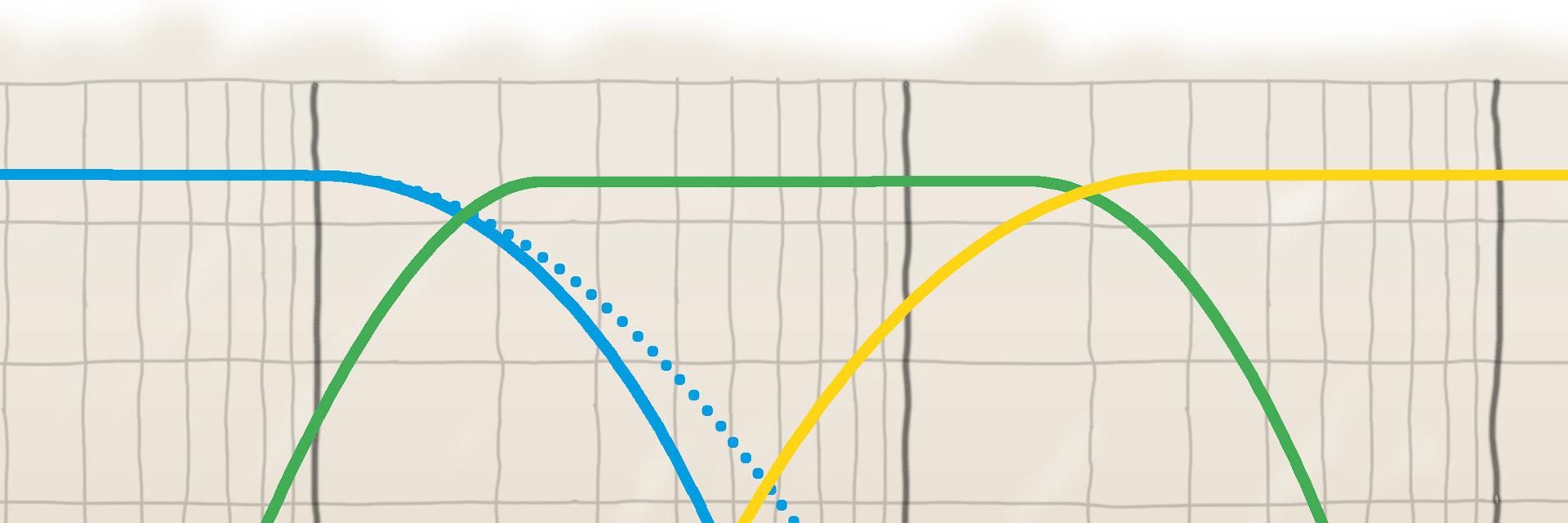
Another important parameter of the filter function is the damping – the so-called quality factor or Q-value, which describes how smoothly or abruptly the transfer function approaches its slope in the range of the crossover frequency. At low Q values, the transition occurs gradually over a wide frequency range; at higher Q values, it occurs correspondingly faster.
Thus, a crossover for a two-way loudspeaker consists of a high-pass filter in front of the tweeter and a low-pass filter in front of the mid-bass driver. Each of the two drivers takes care of the frequency range it handles best, and thus everything should be fine. Unfortunately, however, this is not the case: in fact, the filtering delays the signal by 90 degrees per filter order – so it’s not just a matter of achieving a smooth frequency response, but also of getting the phase behavior well enough under control to produce a predictable, even and time-correct dispersion pattern. In choosing the type of filter, one has “the choice between cholera and plague,” as Fink describes.
Smooth frequency response …
The two most common filter types are Butterworth and Linkwitz-Riley filters, which differ not only in filter order but also in their quality factor: Linkwitz-Riley filters are even-order filters with a Q value of 0.5, which means that at the crossover frequency, both ways – mid/bass and treble – are attenuated by 6 dB each compared to the input level. Second-order Linkwitz-Riley filters produce a phase offset of 180 degrees, which would cause the frequenzy branches to cancel each other out. The solution is quite simple: simply reverse the polarity of one of the two drivers, causing the phase to rotate another 180 degrees and thus arrive back at zero – albeit delayed by one wavelength. With a fourth-order filter, this problem solves itself by a 360-degree phase rotation. The time delay is not a significant problem as long as the crossover frequency is reasonably high – at 2.5 kilohertz we’re talking 0.4 milliseconds.
Since the -6 dB at the crossover frequency correspond to half the original signal amplitude, our elementary school math skills tell us that the two branches add up exactly to the correct level – but that’s only true on axis. If the listener moves his head up or down, the phase of the frequency branches shifts in relation to each other. They now no longer add up completely or, in extreme cases, even cancel each other out, causing the level to drop off-axis in the transition range. “Especially at low crossover frequencies – for example, with three-way loudspeakers – this can lead to a rather thin-sounding low end, since less power is radiated into the room overall as a result of the filtering,” Fink elaborates. This can be mitigated with higher filter orders. The steeper filter slopes limit the problem to a narrower frequency band, but this in turn means accepting a greater overall time offset, which can be considerable at low crossover frequencies.
… or uniform dispersion?
Or you decide for a Butterworth filter. This is characterized by an odd order and a Q-value of 0.707. This higher Q-value (one speaks of lower damping) means that the level at the crossover frequency is only 3 dB below the input signal – so in itself one would expect a hump of 3 dB when adding the two branches. However, due to the phase offset by 90 degrees (first order), the levels do not add up completely and a largely smooth frequency response in the crossover region is the result. Since the phase turns towards 0 or 180 degrees as you move your head vertically (depending on whether you move up or down), you end up with a mixture of peaks and troughs that add up to more or less the right amount of radiated power. However, you can probably already see where the problem lies: Off the listening axis, Butterworth filters sometimes behave quite unpredictably and unrefined because of their “skewed” phase behavior, which makes it difficult to achieve an overall balanced frequency response.
“No matter how you do it, you do it wrong,” the cynic may now lament – and indeed, every loudspeaker is ultimately a box of compromises. Perfection doesn’t exist anywhere, however, and when you take a seat in your chair tonight, fire up the system, and press play, you’ll have another opportunity to marvel at how damn well compromises can work when the developers behind them know what they’re doing.
Special thanks to Karl-Heinz Fink.